How to Do Continuity in Calculus as a Piecewise Functions
FINDING CONTINUITY OF PIECEWISE FUNCTIONS
About "Finding Continuity of Piecewise Functions"
Finding Continuity of Piecewise Functions :
Here we are going to how to find out the point of discontinuity for a piecewise function.
Finding Continuity of Piecewise Functions - Examples
Question 1 :
A function f is defined as follows :
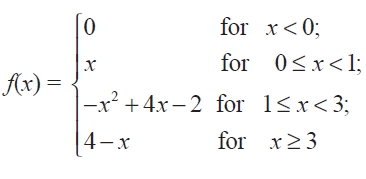
Is the function continuous?
Solution :
(i) First let us check whether the piece wise function is continuous at x = 0.
For the values of x lesser than 0, we have to select the function f(x) = 0.
lim x->0- f(x) =limx->0- 0
= 0 -------(1)
For the values of x greater than 0, we have to select the function f(x) = x.
limx->0+ f(x) = limx->0+ x
= 0 -------(2)
lim x->0 - f(x) = lim x->0 + f(x)
Hence the function is continuous at x = 0.
(ii) Let us check whether the piece wise function is continuous at x = 1.
For the values of x lesser than 1, we have to select the function f(x) = x.
limx->1- f(x) = limx->1- 0
= 1 -------(1)
For the values of x greater than 1, we have to select the function f(x) = -x2 + 4x - 2.
limx->1+ f(x) = limx->1+ (-x2 + 4x - 2)
= -12 + 4(1) - 2
= -1 + 4 - 2
= 1 -------(2)
lim x->1 - f(x) = lim x->1 + f(x)
Hence the function is continuous at x = 1.
(iii) Let us check whether the piece wise function is continuous at x = 3.
For the values of x lesser than 3, we have to select the function f(x) = -x2 + 4x - 2.
limx->3- f(x) = limx->3- -x2 + 4x - 2
= -32 + 4(3) - 2
= -9 + 12 - 2
= -11 + 12
= 1 -------(1)
For the values of x greater than 3, we have to select the function f(x) = 4 - x
limx->3+ f(x) = limx->3+ 4 - x
= 4 - 3
= 1 -------(2)
lim x->3 - f(x) = lim x->3 + f(x)
Hence the function is continuous at x = 3.
Question 2 :
Find the points of discontinuity of the function f, where
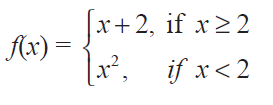
Solution :
For the values of x greater than 2, we have to select the function x + 2.
limx->2- f(x) = limx->2- x + 2
= 2 + 2
= 4 -------(1)
For the values of x lesser than 2, we have to select the function x2.
limx->2+ f(x) = limx->2+ x2
= 22
= 4-------(2)
lim x->2 - f(x) = lim x->2 + f(x)
The function is continuous at x = 2.
Hence the given piecewise function is continuous for all x ∈ R.
Question 3 :
Find the points of discontinuity of the function f, where
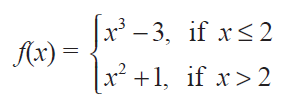
Solution :
For the values of x greater than 2, we have to select the function x2 + 1
limx->2- f(x) = limx->2- x2 + 1
= 2 2 + 1
= 5 -------(1)
For the values of x lesser than 2, we have to select the function x3 - 3.
limx->2+ f(x) = limx->2+ x3- 3
= 23 - 3
= 8 - 3
= 5-------(2)
lim x->2 - f(x) = lim x->2 + f(x)
The function is continuous at x = 2.
Hence the given piecewise function is continuous for allx ∈ R.
Question 4 :
Find the points of discontinuity of the function f, where
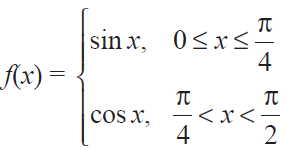
Solution :
Here we are going to check the continuity between 0 andπ/2.
For the values of x lesser than or equal toπ/4 , we have to choose the function sin x.
limx-> π/4- f(x) = limx-> π/4 - sin x
= sin (π/4)
= 1/ √2
For the values of x greater thanπ/4, we have to choose the function cos x .
limx-> π/4+ f(x) = limx-> π/4 + cos x
= cos (π/4)
= 1/ √2
The function is continuous for all x ∈ [0, π/2).

After having gone through the stuff given above, we hope that the students would have understood, " Finding Continuity of Piecewise Functions"
Apart from the stuff given in " Finding Continuity of Piecewise Functions" , if you need any other stuff in math, please use our google custom search here.
Kindly mail your feedback tov4formath@gmail.com
We always appreciate your feedback.
© All rights reserved. onlinemath4all.com
mcdonelldiferathe.blogspot.com
Source: https://www.onlinemath4all.com/finding-continuity-of-piecewise-functions.html